
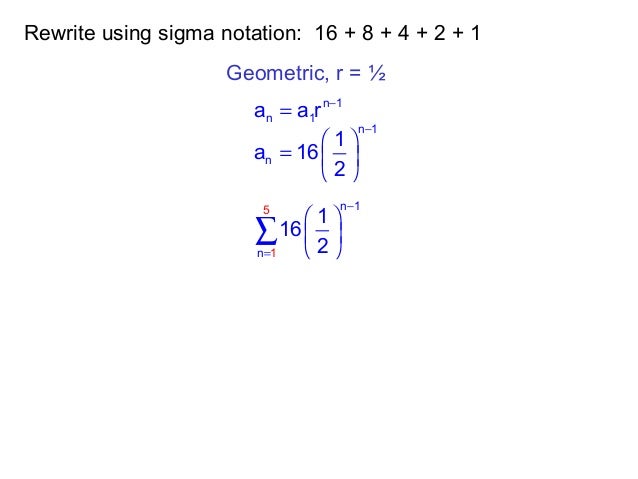
The constant ratio of the geometric sequence 3/5,3/2,15/4,75/8, is. So for example, this is a geometric sequence. A series, the most conventional use of the word series, means a sum of a sequence. However, the intersection of infinitely many infinite arithmetic progressions might be a single number rather than itself being an infinite progression. Let S n be the sum of a geometric sequence with n terms. And you might even see a geometric series. If each pair of progressions in a family of doubly infinite arithmetic progressions have a non-empty intersection, then there exists a number common to all of them that is, infinite arithmetic progressions form a Helly family. In other words, if you keep adding together the terms of the sequence forever, you will get a finite value. In geometric progressions where r < 1 (in other words where r is less than 1 and greater than 1), the sum of the sequence as n tends to infinity approaches a value. The intersection of any two doubly infinite arithmetic progressions is either empty or another arithmetic progression, which can be found using the Chinese remainder theorem. The sum to infinity of a geometric progression. The formula is very similar to the standard deviation of a discrete uniform distribution. If the initial term of an arithmetic progression is a 1 is the common difference between terms. A geometric sequence is a type of sequence such that when.
#Arithmetic and geometric sequences formulas sum series
An infinite geometric series converges (has a sum).
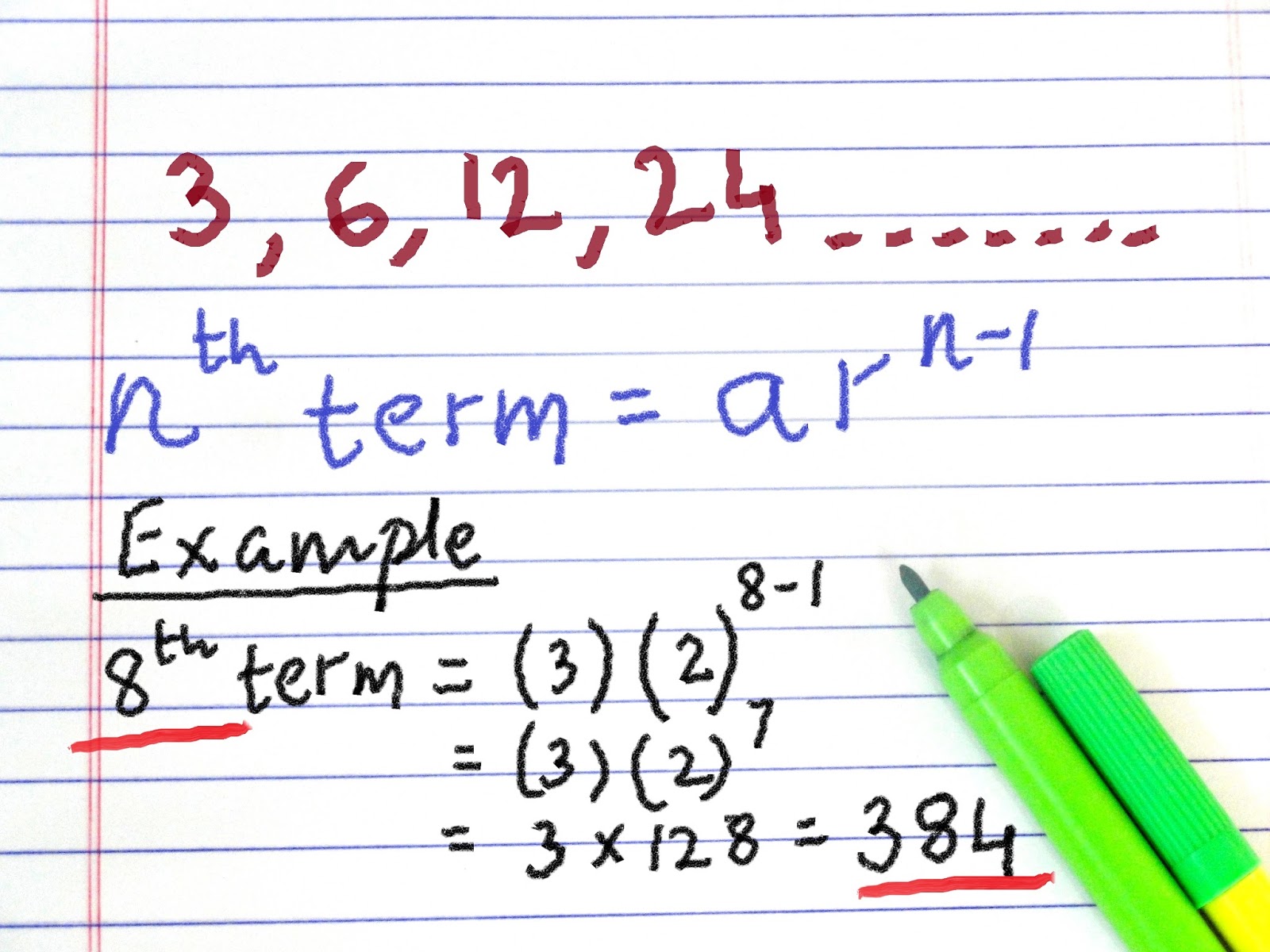
is an arithmetic progression with a common difference of 2. Remember, a sequence is simply a list of numbers while a series is the sum of the list of numbers. The two most straightforward sequences we can work with are arithmetic and geometric sequences. When 1 < r < 1 you can use the formula S a 1 1 r to find the sum of the infinite geometric series. The constant difference is called common difference of that arithmetic progression. The sum of arithmetic sequence with first term a (or) a 1 and common difference d is denoted by S n and can be calculated by one of the two formulas. An arithmetic progression or arithmetic sequence ( AP) is a sequence of numbers such that the difference from any succeeding term to its preceding term remains constant throughout the sequence. I like to compare sequences to relations or functions we learned about in the Algebraic Functions section.Think of the (x)-part of the relation (the independent variable) as the numbers in a row that represent each part of the sequence these typically start at 1 (one).


 0 kommentar(er)
0 kommentar(er)
